Quantitative Aptitude Quiz For IBPS | RBI | SBI | NABARD | 30-06-2022

 

Dear Readers,

Mahendras has started special quizzes for IBPS  | RBI  | SBI  | NABARD  | so that you can practice more and more to crack the examination. This IBPS  | RBI  | SBI  | NABARD  | Exam special quiz series will mold your preparations in the right direction and the regular practice of these quizzes will be really very helpful in scoring good marks in the Examination. Here we are providing you the important question of reasoning ability for the for the the IBPS  | RBI  | SBI  | NABARD  | .

Q-1 A box contains 4 white balls, 5 black balls and 7 green balls. In how many ways can 4 balls be drawn from the box, if at least one white ball, one black ball and one green ball is to be included in a draw?

01. 910

02. 920

03. 890

04. 940

05. 840

एक बॉक्स में 4 सफ़ेद गेंदें, 5 काली गेंदें और 7 हरी गेंदें हैं | कितने तरीकों से 4 गेंदें बॉक्स से निकाली जा सकती हैं, यदि एक बार में कम से कम एक सफ़ेद गेंद, एक काली गेंद और एक हरी गेंद को शामिल किया जाता है ?

01. 910

02. 920

03. 890

04. 940

05. 840

Q-2 Shivam, Divya, Eva and Gagan are employed to finish a work. Eva takes 4 days less than Shivam to finish the work while Gagan takes 1/4th of the time taken by Divya to finish the work. Together they can finish the work in 1 day. Due to some illness Gagan was not able to work, so Shivam, Divya and Eva started working together without Gagan. After 1 day, Eva left and the work was finished in 2 more days. Find the number of days in which Eva can finish the work alone.

01. 4 days

02. 2 days

03. 8 days

04. 12 days

05. None of these

शिवम, दिव्या, ईवा और गगन किसी कार्य को पूरा करने के लिए कार्यरत हैं। ईवा को कार्य को पूरा करने में शिवम से 4 दिन कम समय लगता है जबकि गगन को कार्य समाप्त करने में दिव्या द्वारा लिये गये समय का 1 / 4 वाँ भाग समय लगता है । एकसाथ वे 1 दिन में काम खत्म कर सकते हैं। कुछ बीमारी के कारण गगन काम करने में सक्षम नहीं था, इसलिए शिवम, दिव्या और ईवा ने गगन के बिना एक साथ काम करना शुरू कर दिया। 1 दिन के बाद, ईवा ने काम छोड़ दिया और 2 और दिनों में काम खत्म हो गया। दिनों की संख्या ज्ञात कीजिये जिसमें ईवा अकेले कार्य समाप्त कर सकता है।

01. 4 दिन

02. 2 दिन

03. 8 दिन

04. 12 दिन

05. इनमें से कोई नहीं

Q-3 In a class there are 15 boys and 10 girls. Three students are selected at random. The difference between the probability that 2 boys and 1 girl are selected compared to 1 boy and 2 girls are selected is:

01. 23/78

02. 19/88

03. 7/46

04. 4/23

05. 15/92

एक कक्षा में 15 लड़के और 10 लडकियां हैं | तीन छात्रों की यादृच्छया चुना जाता है | 2 लड़कों और 1 लड़के के चुनने की प्रायिकता और 1 लड़के और 2 लड़कियों के चुनने की प्रायिकता में क्या अन्तर है :

01. 23/78

02. 19/88

03. 7/46

04. 4/23

05. 15/92

Q-4 A bottle contains a mixture of two liquids A and B in the ratio 5: 3. If 6 litres of the mixture is replaced by 6 litres of liquid B, then the ratio of the two liquids becomes 5: 9. How much of the liquid A was there in the bottle? (Litres)

01. 8

02. 8.25

03. 8.75

04. 9.25

05. 9.75

एक बोतल में 5: 3 के अनुपात में दो तरल पदार्थ A और B का मिश्रण है। यदि 6 लीटर मिश्रण को 6 लीटर तरल B से प्रतिस्थापित किया जाता है, तो दोनों तरल पदार्थों का अनुपात 5: 9 हो जाता है। तरल A का कितना भाग (लीटर) बोतल में था?

01. 8

02. 8.25

03. 8.75

04. 9.25

05. 9.75

Q-5 Rakesh adds 12% of his salary in PPF. 3/8 of the remaining salary is spent in clothes. Difference between PPF and clothes expanses is Rs. 10500. Remaining in house rent and others. If house rent expenses is Rs. 1500 less than expanses in others, then what is the house rent expenses?

01. 17000

02. 10000

03. 9000

04. 13000

05. None of these

पीपीएफ में राकेश अपने वेतन का 12% जोड़ते हैं । शेष वेतन का 3/8 भाग कपड़े में खर्च किया जाता है। पीपीएफ और कपड़े के खर्च के बीच अंतर रु. 10500 है । शेष घर के किराए और अन्य में । यदि घर का किराये का खर्च दूसरे खर्चों से रु. 1500 कम है, तो घर का किराये का खर्च क्या है?

01. 17000

02. 10000

03. 9000

04. 13000

05. इनमें से कोई नहीं

Q-6 A bag contains 5 red balls, 4 green balls and 3 blue balls. Three balls are drawn at random, what is the probability that at least 2 are red?

01. 7/22

02. 8/13

03. 4/11

04. 7/11

05. 5/11

एक बैग में 5 लाल गेंदें, 4 हरी गेंदें और 3 नीली गेंदें हैं। तीन गेंदों को यादृच्छिक रूप से निकाला जाता है, क्या संभावना है कि निकाली गयी गेंदों में से कम से कम 2 लाल हैं?

01. 7/22

02. 8/13

03. 4/11

04. 7/11

05. 5/11

Q-7 A merchant dealer gives successive discounts of 10% and 20% in that order and makes a profit of 20%. What will be the profit if he offers a single flat discount of 30%?

01. 20%

02. 16.67%

03. 18%

04. 14%

05. None of these

एक थोक व्यापारी 10% और 20% की दो छूट देकर भी 20% का लाभ प्राप्त करता है | लाभ क्या होगा यदि वह 30% की एकल छूट देता है ?

01. 20%

02. 16.67%

03. 18%

04. 14%

05. इनमें से कोई नहीं

Q-8 X, Y and Z invested Rs. 14000 in total in a business. X invested Rs. 3120 more than Y and Z invested Rs. 1720 less than Y. The total profit was Rs. 35000. Find the share of Z.

01. Rs. 8100

02. Rs. 6200

03. Rs. 7280

04. Rs. 7040

05. Rs. 6800

X, Y और Z ने एक व्यवसाय में कुल मिलाकर रु. 14000 निवेश किये । X ने Y से रु. 3120 अधिक और Z ने Y से रु. 1720 कम निवेश किया । कुल लाभ रु. 35000 था । Z का हिस्सा ज्ञात कीजिए।

01. रु. 8100

02. रु. 6200

03. रु. 7280

04. रु. 7040

05. रु. 6800

Q-9 Two trains A and B start moving at the same time from P and Q location which are 80 km apart. If the two trains travel in opposite direction from P and Q respectively then they meet each other in 1 hour and if they in the same direction from P and Q respectively then they meet after 8 hours. Find the speed of the train A.

01. 40 km/hr

02. 45 km/hr

03. 64 km/hr

04. 58 km/hr

05. 35 km/hr

दो ट्रेनें A और B एक ही समय में P और Q स्थान से चलना शुरू करती हैं जो 80 किमी दूर हैं। यदि दोनों ट्रेनें क्रमशः P और Q से विपरीत दिशा में यात्रा करती हैं तो वे 1 घंटे में एक दूसरे से मिलती हैं और यदि वे क्रमशः P और Q से एक ही दिशा में जाती हैं तो वे 8 घंटे के बाद मिलती हैं । ट्रेन A की गति ज्ञात कीजिए।

01. 40 किमी/घंटा

02. 45 किमी/घंटा

03. 64 किमी/घंटा

04. 58 किमी/घंटा

05. 35 किमी/घंटा

Q-10 A boat can go BA (upstream) in 84 minutes. The same boat when going downstream (AB) takes 9 minutes less than what it would take in still water. How much time will it take to reach from A to B (downstream)?

01. 29 min

02. 37 min

03. 48 min

04. 63 min

05. 83 min

एक नाव 84 मिनट में BA (अपस्ट्रीम) जा सकती है। डाउनस्ट्रीम (AB) जाते समय उसी नाव को स्थिर जल में लिए जाने वाले समय से 9 मिनट कम समय लगता है । A से B (डाउनस्ट्रीम) तक पहुंचने में कितना समय लगेगा?

01. 29 मिनट

02. 37 मिनट

03. 48 मिनट

04. 63 मिनट

05. 83 मिनट

Answer:-

Q-1(1)

Total number of balls = 4 + 5 + 7 = 16

Required number of ways = (⁴C₁ × ⁵C₁ × ⁷C₂) + (⁴C₁ × ⁵C₂ × ⁷C₁) + (⁴C₂ × ⁵C₁ × ⁷C₁)

= (4 × 5 × 21) + (4 × 10 × 7) + (6 × 5 × 7)

= 420 + 280 + 210 = 910

कुल गेंदों की संख्या = 4 + 5 + 7 = 16

अभीष्ट प्रकारों की संख्या = (⁴C₁ × ⁵C₁ × ⁷C₂) + (⁴C₁ × ⁵C₂ × ⁷C₁) + (⁴C₂ × ⁵C₁ × ⁷C₁)

= (4 × 5 × 21) + (4 × 10 × 7) + (6 × 5 × 7)

= 420 + 280 + 210 = 910

Q-2(1)

Let Shivam takes x days to complete the work and Divya takes y days to complete the work.

Then number of days taken by Eva to complete the work = x – 4

and number of days taken by Gagan to complete the work = y/ 4

According to the question,

… (I)

and

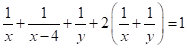… (II)

Now, equation (I) – (II), we get

x = y

Now, from (I), we get

6 (x – 4) + x = x (x – 4)

6x – 24 + x = x2 – 4x

x² – 11x – 24 = 0

x = 8, 3 (inadmissible)

Required number of days = 8 – 4 = 4 days

माना शिवम् कार्य को पूरा करने के लिए x दिन लेता है और कार्य को पूरा करने के लिए दिव्या y दिन लेती है ।

कार्य को पूरा करने के लिए ईवा द्वारा लिए गए दिनों की संख्या = x – 4

और गगन द्वारा कार्य को पूरा करने में लिए गए दिनों की संख्या = y/ 4

प्रश्नानुसार,

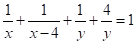… (I)

और

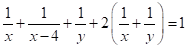… (II)

अब, समीकरण (I) – (II), हम प्राप्त करते हैं

x = y

अब, (I) से, हम प्राप्त करते हैं

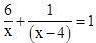
6 (x – 4) + x = x (x – 4)

6x – 24 + x = x2 – 4x

x²  – 11x – 24 = 0

x = 8, 3 (अमान्य)

अभीष्ट दिनों की संख्या = 8 – 4 = 4 दिन

Q-3(5)

P (2 boys, 1 girl)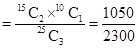

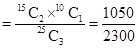
P (2 girls, 1 boy) =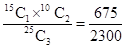

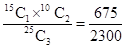
Difference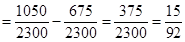

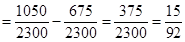
P (2 लड़के, 1 लड़की)

P (2 लडकियां, 1 लड़का) =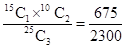

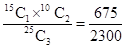
अन्तर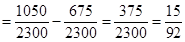

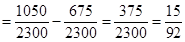
Q-4(3)

According to the question,

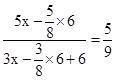
(20x – 15) × 9 = (12x – 9 + 24) × 5

180x – 135 = 60x – 45 + 120

x = 7/4

Liquid A in bottle 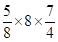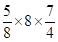= = 8.75

प्रश्नानुसार,

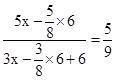
(20x – 15) × 9 = (12x – 9 + 24) × 5

180x – 135 = 60x – 45 + 120

x = 7/4

बोतल में तरल A = = 8.75

Q-5(4)

Let total salary be Rs. 100.

According to the question,

= 10500

33 – 12 = 10500

21 = 10500

1 = 500

100 = 50000

Now, x – 1500 + x = 55 × 500

2x = 27500 + 1500

2x = 29000

x = 14500

Expanse on house rent = 14500 – 1500 = 13000

माना कुल वेतन रु. 100 है ।

प्रश्नानुसार,

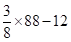= 10500

33 – 12 = 10500

21 = 10500

1 = 500

100 = 50000

अब, x – 1500 + x = 55 × 500

2x = 27500 + 1500

2x = 29000

x = 14500

घर के खर्च पर किराया = 14500 – 1500 = 13000

Q-6(3)

Required probability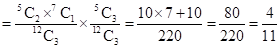

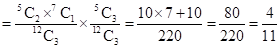
अभीष्ट प्रायिकता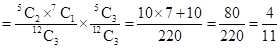

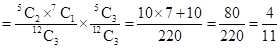
Q-7(2)

Let marked price be 100.

SP with 10%, 20% discount = 80% of (90% of 100) = 72.

Profit = 20%

So, CP = 72 ÷ 1.20 = Rs. 60

By offering a flat discount of 30%, sales price = 70% of 100 = 70

Profit = [(70 - 60) ×100] ÷ 60 = 16.67%

माना अंकित मूल्य 100 रु. है |

10%, 20% की छूट के बाद विक्रय मूल्य = (100 का 90%) का 80% = 72.

लाभ = 20%

अतः, क्रय मूल्य = 72 ÷ 1.20 = रु.60

30% की एकल छूट देने के बाद विक्रय मूल्य = 100 का 70% = 70

लाभ = [(70 - 60) ×100] ÷ 60 = 16.67%

Q-8(2)

x + 3120 + x + x – 1720 = 14000

3x = 12600

x = 4200

Required ratio between equivalent capitals of X, Y and Z

= (4200 + 3120): (4200): (4200 – 1720) = 7320: 4200: 2480

= 183: 105: 62

Share of Z 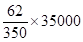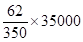= = Rs. 6200

x + 3120 + x + x – 1720 = 14000

3x = 12600

x = 4200

X, Y और Z की समतुल्य पूंजियों में अनुपात

= (4200 + 3120): (4200): (4200 – 1720) = 7320: 4200: 2480

= 183: 105: 62

Z का हिस्सा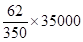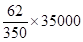 = = Rs. 6200

Q-9(2)

Let the speed of the train A and train B be x kmph and y kmph respectively.

According to the question,

80 = (x + y) × 1 … (I)

80 = (x – y) × 8 … (II)

x – y = 10

x + y = 80

On solving above,

x = 45 kmph

माना ट्रेन A और ट्रेन B की चाल क्रमशः x किमी/घंटा और y किमी/घंटा है ।

प्रश्नानुसार,

80 = (x + y) × 1 … (I)

80 = (x – y) × 8 … (II)

x – y = 10

x + y = 80

उपर्युक्त को हल करने पर,

x = 45 किमी/घंटा

Q-10(4)

Let x be the speed of the boat in still water and y be the speed of the stream.

According to the question,

D = (x – y) × 84 … (I)

D = (x + y) × t … (II)

D = x (t + 9) … (III)

From (I) and (II), we get

2x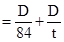

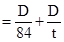
On putting the value of x from equation (III), we get

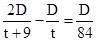
On solving above, we get

t = 63 minutes or 12 minutes

माना नाव की स्थिर जल में चाल x और धारा की चाल y है ।

प्रश्नानुसार,

D = (x – y) × 84 … (I)

D = (x + y) × t … (II)

D = x (t + 9) … (III)

(I) और (II) से, हम प्राप्त करते हैं

2x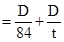

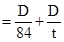
समीकरण (III) से x का मान रखने पर, हम प्राप्त करते हैं

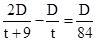
उपर्युक्त को हल करने पर, हम प्राप्त करते हैं

t = 63 मिनट या 12 मिनट