Dear Readers,
As SSC MTS | CHSL notification is out and candidates have started their preparation for this exam. Mahendras also has started special quizzes for this examination. This series of quizzes are based on the latest pattern of the SSC MTS | CHSL examination. Regular practice of the questions included in the quizzes will boost up your preparations and it will be very helpful in scoring good marks in the examination.
Q-1
The perimeter and length of a rectangle are 40 m and 12 m respectively. Its area will be -
एक आयत का परिमाप और लम्बाई क्रमशः 40 मी. और 12 मी. हैं। इसका क्षेत्रफल होगा -
A. 120 m.2
B. 96 m.2
C. 72 m.2
D. 36 m.2
Q-2
In a right angled triangle, the circumcentre of the triangle lies -
एक समकोण त्रिभुज में, त्रिभुज का परिकेंद्र स्थित होता है -
A. inside the triangle/त्रिभुज के अन्दर
B. on midpoint of the hypotenuse/कर्ण के मध्य बिंदु पर
C. outside the triangle/ त्रिभुज के बाहर
D. on one vertex/एक शीर्ष पर
Q-3
Two circles having radii 'a' units intersect each other in such a way that each of them passes through the centre of the other. Then find the length of their common chord.
दो वृत्तों जिनकी त्रिज्याएँ 'a' मात्रक हैं, एक दूसरे को इस तरह से प्रतिच्छेदित करते हैं कि उनमें से प्रत्येक दूसरे के केंद्र से होकर जाता है। तो उनकी उभयनिष्ठ जीवा की लम्बाई ज्ञात कीजिये।
A. 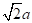 units/मात्रक
units/मात्रक
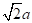 units/मात्रक
units/मात्रकB. 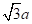 units/मात्रक
units/मात्रक
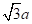 units/मात्रक
units/मात्रकC. 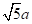 units/मात्रक
units/मात्रक
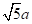 units/मात्रक
units/मात्रकD. a units/मात्रक
Q-4
If 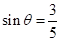 and
and 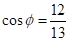 what is the value of
what is the value of 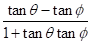 ; it is given that θ and
; it is given that θ and 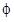 are acute angles?
are acute angles?
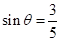 and
and 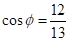 what is the value of
what is the value of 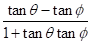 ; it is given that θ and
; it is given that θ and 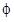 are acute angles?
are acute angles?यदि 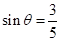 और
और 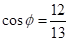 है ।
है ।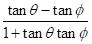 का मान क्या होगा जबकि यह ज्ञात है कि θ और
का मान क्या होगा जबकि यह ज्ञात है कि θ और  न्यूनकोण हैं?
न्यूनकोण हैं?
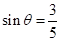 और
और 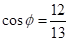 है ।
है ।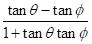 का मान क्या होगा जबकि यह ज्ञात है कि θ और
का मान क्या होगा जबकि यह ज्ञात है कि θ और  न्यूनकोण हैं?
न्यूनकोण हैं?A. 15/63
B. 19/63
C. 17/65
D. 16/63
Q-5
The angle of elevation of the top of a tower from a distance of 100 m from its foot is 300. The height of the tower is -
एक मीनार के पाद से 100 मी. की दूरी पर स्थित एक बिंदु से मीनार के शिखर का उन्नयन कोण 300 मीनार की ऊँचाई है -
A. 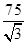 m./मी.
m./मी.
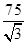 m./मी.
m./मी.B. 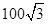 m./मी.
m./मी.
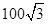 m./मी.
m./मी.C. 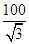 m./मी.
m./मी.
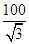 m./मी.
m./मी.D. 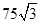 m./मी.
m./मी.
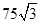 m./मी.
m./मी.Q-6
If 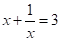 , then find the value of
, then find the value of 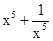 .
.
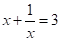 , then find the value of
, then find the value of 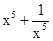 .
.यदि 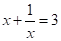 तो
तो  का मान ज्ञात कीजिये।
का मान ज्ञात कीजिये।
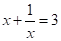 तो
तो  का मान ज्ञात कीजिये।
का मान ज्ञात कीजिये।A. 110
B. 132
C. 122
D. 123
Q-7
If a = b = c. then 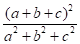 is equal to-
is equal to-
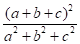 is equal to-
is equal to-यदि a = b = c तो 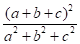 का मान बराबर है -
का मान बराबर है -
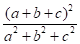 का मान बराबर है -
का मान बराबर है -A. 4
B. 3
C. 2
D. 1
Q-8
A motor boat goes 2 km upstream in a stream flowing at the rate of 3 km per hour and then returns downstream to the starting point in 30 minutes. Find the speed of the motor boat in still water.
एक मोटर बोट 2 किमी. ऊर्ध्वप्रवाह में 3 किमी.प्रति घंटे की चाल से गति करती है और फिर अनुप्रवाह में गति करते हुए मूल बिंदु पर 30 मिनट में पहुँचती है। स्थिर जल में मोटर बोट की चाल ज्ञात कीजिये।
A. 9.5 kmph/किमी./घंटा
B. 8.5 kmph/किमी./घंटा
C. 9 kmph/किमी./घंटा
D. 8 kmph/किमी./घंटा
Q-9
A train running at 7/11of its own speed reached a place in 33 hours. How much time could be saved if the train would run at its own speed?
एक रेलगाड़ी अपनी चाल के 7/11चाल से चलती हुई एक स्थान पर 33 घंटे में पहुँचती है। यदि रेलगाड़ी अपनी वास्तविक चाल से चले तो कितना समय बच सकता है?
A. 11 hours/घंटे
B. 18 hours/घंटे
C. 15 hours/घंटे
D. 14 hours/घंटे
Q-10
Twenty women together can complete a work in 16 days. 16 men together can complete the same work in 15 days. The ratio of the working capacity of a woman to that of a man is -
20 औरतें एक काम को 16 दिनों में पूरा कर सकती हैं। 16 आदमी उसी काम को 15 दिनों में पूरा कर सकते हैं। एक महिला और एक पुरुष की कार्यक्षमता में क्रमशः अनुपात है -
A. 4 : 3
B. 3 : 4
C. 3 : 5
D. 5 : 4
ANSWER:-
Q-1(2)
Length of the rectangle/आयत की लम्बाई = 12 m./मी.
Perimeter of the rectangle/आयत का परिमाप = 40 m./मी.
2 (12 + x) = 40
12 + x = 20
x = 8 m.
Its area/इसका क्षेत्रफल = 12 × 8 = 96 m.2/मी.2
Q-2(2)
on midpoint of the hypotenuse/कर्ण के मध्य बिंदु पर
Q-3(2)
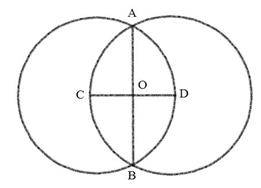
OC = OD = (a/2) units/मात्रक
AC = a units/मात्रक
In ΔAOC/ ΔAOC में
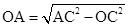
= 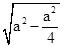 =
= 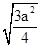 =
= 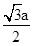
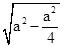 =
= 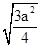 =
= 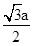
AB = 2 × OA = 2 × 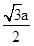
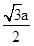
= 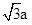
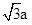
Q-4(2)
(16/63)
Q-5(3)
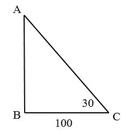
AB = tower/मीनार = h metre/मीटर
BC = 100 metre/मीटर
∠ACB = 300
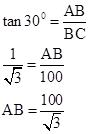
Q-6(4)
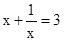
On squaring both the sides/दोनों ओर वर्ग करने पर,
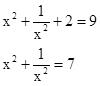 ...(I)
...(I)Again/पुनः,
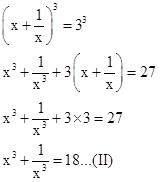
On multiplying (I) and (II), we get/(I) और (II) को गुणा करने पर, हम प्राप्त करते हैं
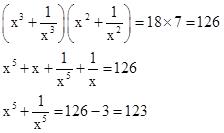
Q-7(2)
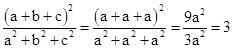
Q-8(3)
Let speed of the boat in still water is x km/h /माना नाव की स्थिर जल में चाल x किमी. /घंटा है
According to the question/प्रश्नानुसार,
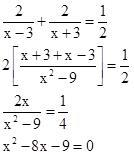
On solving above equation, we get/उपर्युक्त समीकरण को हल करने पर, हम प्राप्त करते हैं
x = 9
Q-9(1)
Since the train runs at 7/11 of its own speed, the time it takes is 11/7 of its usual speed.
Let the usual time taken be t hours.
Then we can write,
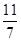 t = 33
t = 33t = 21 hours
Hence time saved = 33 - 21 = 11 hours
Q-10(2)
20 W × 16 = 16 M × 15
4W = 3M
M : W = 4 : 3
W : M = 3 : 4







0 comments:
Post a Comment
MAHENDRA GURU